Moment Generating Function Of A Binomial Distribution - Moment generating functions definition 2.3.6. Moment generating functions (mgfs) are an essential tool in probability and statistics, providing a compact and efficient. The moment generating function (mgf) of a random variable x is mx(t) = e(etx) = (åx e txf.
Moment generating functions definition 2.3.6. The moment generating function (mgf) of a random variable x is mx(t) = e(etx) = (åx e txf. Moment generating functions (mgfs) are an essential tool in probability and statistics, providing a compact and efficient.
Moment generating functions definition 2.3.6. Moment generating functions (mgfs) are an essential tool in probability and statistics, providing a compact and efficient. The moment generating function (mgf) of a random variable x is mx(t) = e(etx) = (åx e txf.
Moment Generating Functions ppt download
Moment generating functions definition 2.3.6. The moment generating function (mgf) of a random variable x is mx(t) = e(etx) = (åx e txf. Moment generating functions (mgfs) are an essential tool in probability and statistics, providing a compact and efficient.
Moment Generating Functions 8 MGF of binomial mean YouTube
Moment generating functions definition 2.3.6. Moment generating functions (mgfs) are an essential tool in probability and statistics, providing a compact and efficient. The moment generating function (mgf) of a random variable x is mx(t) = e(etx) = (åx e txf.
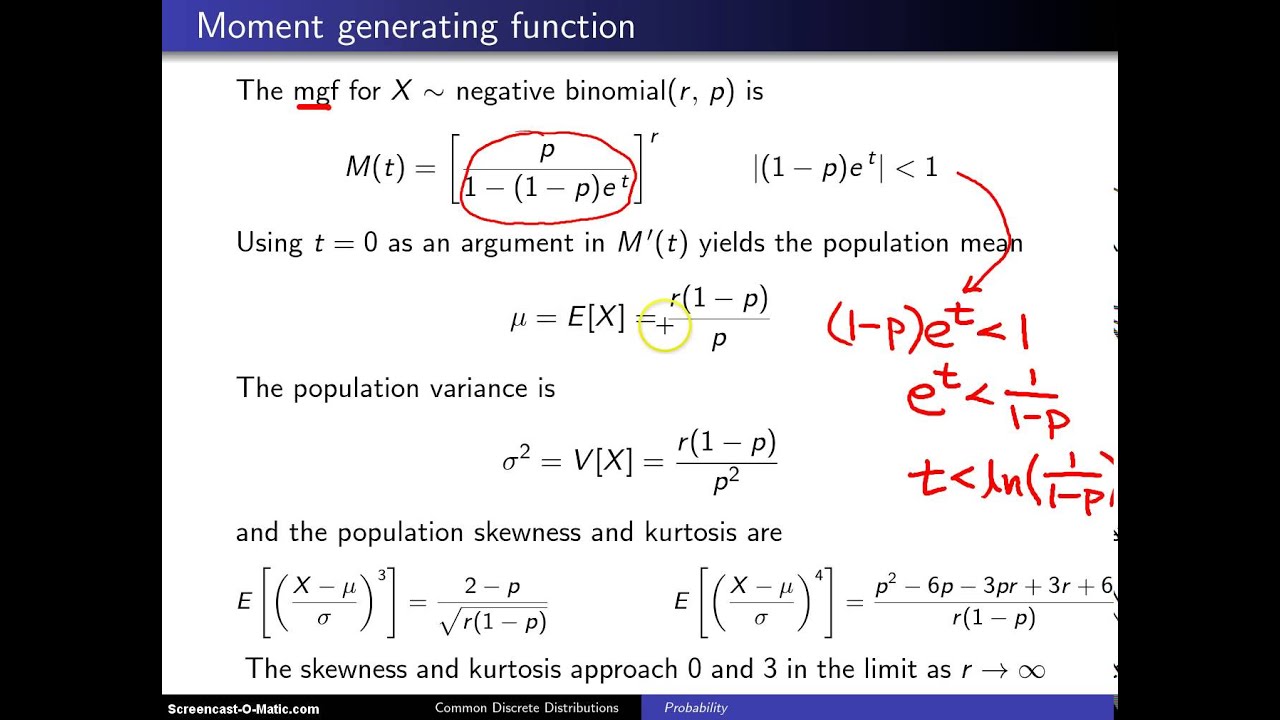
Negative binomial distribution
The moment generating function (mgf) of a random variable x is mx(t) = e(etx) = (åx e txf. Moment generating functions definition 2.3.6. Moment generating functions (mgfs) are an essential tool in probability and statistics, providing a compact and efficient.
Binomial Distribution Derivation of Mean, Variance & Moment
Moment generating functions definition 2.3.6. Moment generating functions (mgfs) are an essential tool in probability and statistics, providing a compact and efficient. The moment generating function (mgf) of a random variable x is mx(t) = e(etx) = (åx e txf.
What is Moment Generating Functions (MGF)?
Moment generating functions definition 2.3.6. The moment generating function (mgf) of a random variable x is mx(t) = e(etx) = (åx e txf. Moment generating functions (mgfs) are an essential tool in probability and statistics, providing a compact and efficient.
PPT Moment Generating Functions PowerPoint Presentation, free
The moment generating function (mgf) of a random variable x is mx(t) = e(etx) = (åx e txf. Moment generating functions definition 2.3.6. Moment generating functions (mgfs) are an essential tool in probability and statistics, providing a compact and efficient.
[Math] Deriving the moment generating function of the negative binomial
Moment generating functions definition 2.3.6. The moment generating function (mgf) of a random variable x is mx(t) = e(etx) = (åx e txf. Moment generating functions (mgfs) are an essential tool in probability and statistics, providing a compact and efficient.
PPT Moment Generating Functions PowerPoint Presentation, free
The moment generating function (mgf) of a random variable x is mx(t) = e(etx) = (åx e txf. Moment generating functions definition 2.3.6. Moment generating functions (mgfs) are an essential tool in probability and statistics, providing a compact and efficient.
Negative binomial moment generating function YouTube
The moment generating function (mgf) of a random variable x is mx(t) = e(etx) = (åx e txf. Moment generating functions definition 2.3.6. Moment generating functions (mgfs) are an essential tool in probability and statistics, providing a compact and efficient.
SOLUTION NU Math 206 Lecture Moment generating function Bernoulli
Moment generating functions (mgfs) are an essential tool in probability and statistics, providing a compact and efficient. The moment generating function (mgf) of a random variable x is mx(t) = e(etx) = (åx e txf. Moment generating functions definition 2.3.6.
The Moment Generating Function (Mgf) Of A Random Variable X Is Mx(T) = E(Etx) = (Åx E Txf.
Moment generating functions (mgfs) are an essential tool in probability and statistics, providing a compact and efficient. Moment generating functions definition 2.3.6.
.jpg)



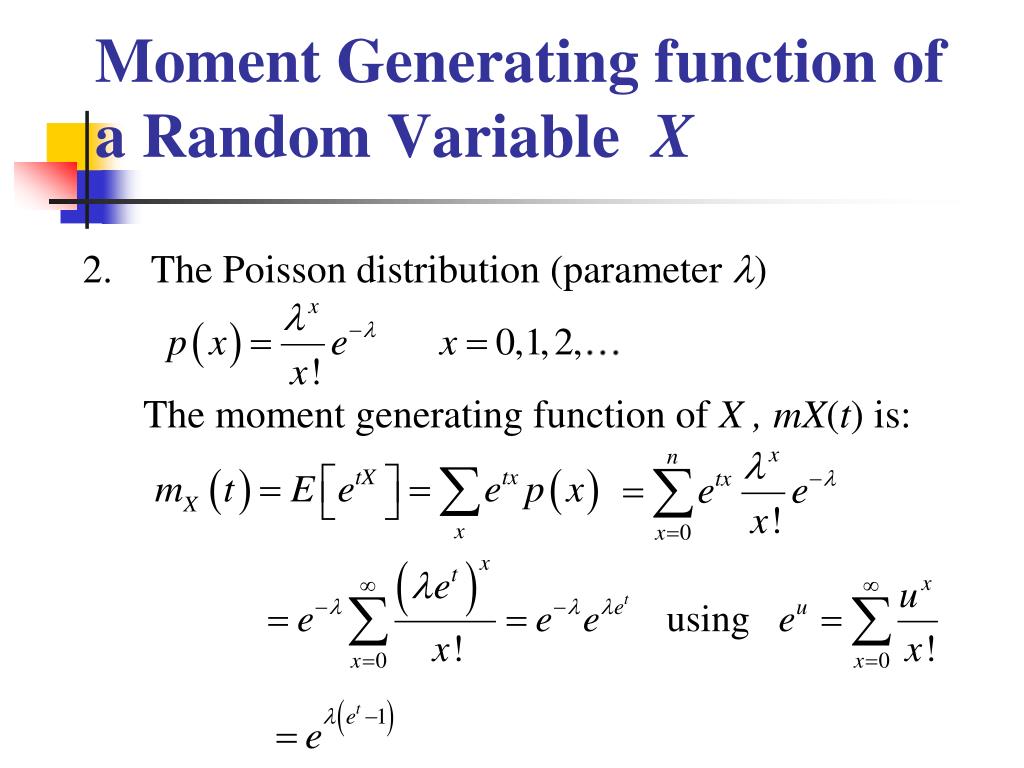
![[Math] Deriving the moment generating function of the negative binomial](https://i.stack.imgur.com/EGd8a.jpg)